
Immediate response - R + GTP + Effector - serpentine structure that spans cell membrane 7 timesĭelayed response - located on DNA in cell nucleus - stimulate transcription of mRNA to make protein - only activated by lipid soluble ligands Immediate response - receptor spans membrane - binding of drug opens channel so specific ions can go through (direction depends on ) Immediate response - receptor spans membrane - binding of drug causes increased catalytic activity on other side of membrane The amount of drug we must give to elicit an effect - a potent drug produces its effects at low doses (potency isn't that important)Ĭhemicals that produce effects by interacting with other chemicals - drugs cannot give cells new functionsĪny functional macromolecule in a cell to which a drug binds to produce its effectsĢ) Receptor activity regulated by endogenous compoundsģ) Drug binding either mimics or blocks action of endogenous molecules (doesn't create new actions) The largest effect that a drug can produce - match the intensity of the response with the patient's need (e.g. Relationship between the size of an administered dose & the intensity of the response produced - usually graded (gets more intense with higher dose)ģ) How much we need to increase the dosage to produce the desired increase in response The study of the biochemical & physiologic effects of drugs & the molecular mechanisms by which those effects are produced (what drugs do to the body & how they do it) "Fitting of logistic S-curves (sigmoids) to data using SegRegA".Properties of the sigmoid, including how it can shift along axes and how its domain may be transformed.) "Continuous output, the sigmoid function". In particular see "Chapter 4: Artificial Neural Networks" (in particular pp. 96–97) where Mitchell uses the word "logistic function" and the "sigmoid function" synonymously – this function he also calls the "squashing function" – and the sigmoid (aka logistic) function is used to compress the outputs of the "neurons" in multi-layer neural nets.) "Beating Floating Point at its Own Game: Posit Arithmetic" (PDF). Physical Audio Signal Processing (2010 ed.). "Variational Gaussian process classifiers".
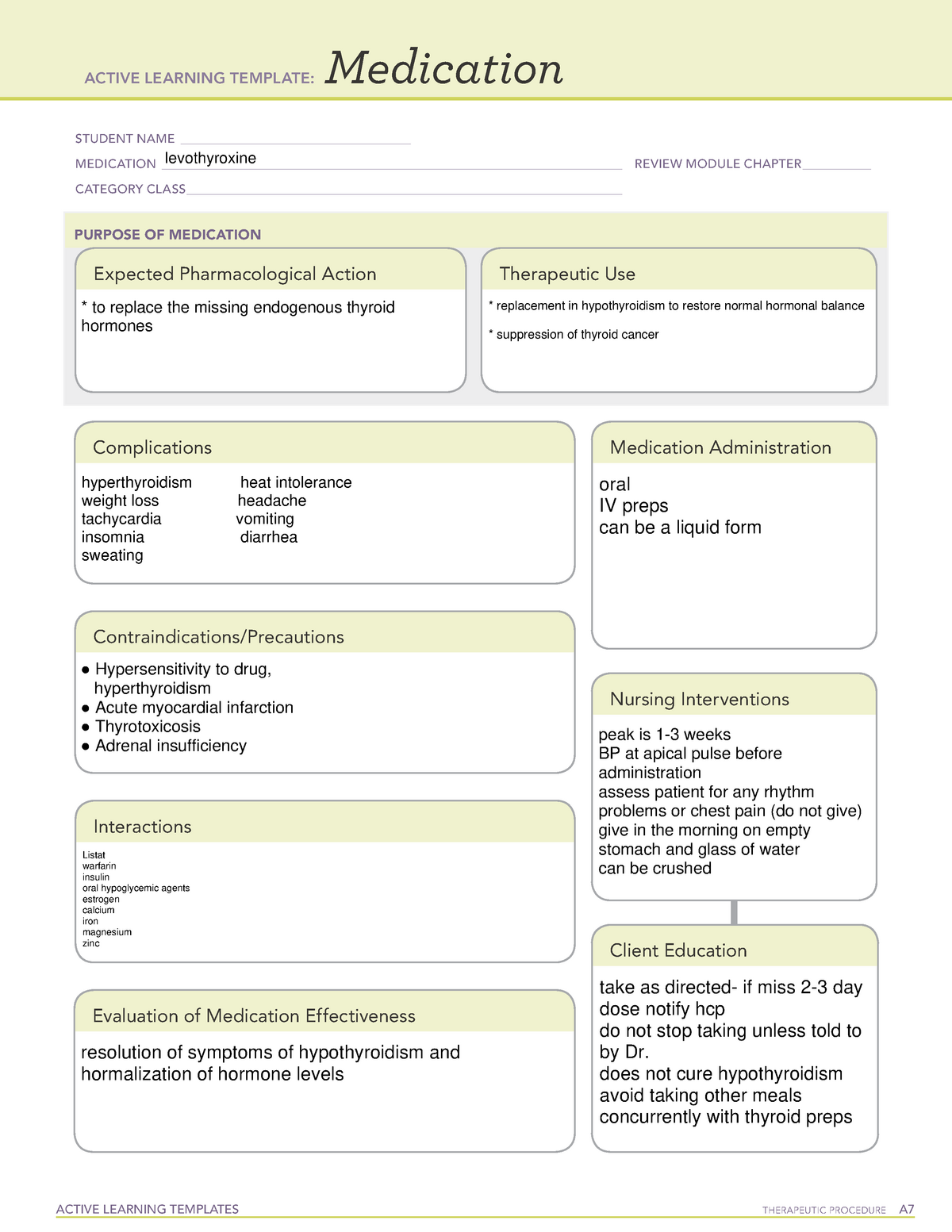
#Study stack pharmacology series#
"Smooth Transition Function in One Dimension | Smooth Transition Function Series Part 1". "Some extensions in continuous methods for immunological correlates of protection". Kensler, Jennifer Coudeville, Laurent Bailleux, Fabrice (). IEEE Transactions on Biomedical Engineering. "Entropic analysis of biological growth models". ^ Ling, Yibei He, Bin (December 1993).From Natural to Artificial Neural Computation. In Mira, José Sandoval, Francisco (eds.). "The influence of the sigmoid function parameters on the speed of backpropagation learning".

The logistic function can be calculated efficiently by utilizing type III Unums. Titration curves between strong acids and strong bases have a sigmoid shape due to the logarithmic nature of the pH scale. In computer graphics and real-time rendering, some of the sigmoid functions are used to blend colors or geometry between two values, smoothly and without visible seams or discontinuities. In biochemistry and pharmacology, the Hill and Hill–Langmuir equations are sigmoid functions. In audio signal processing, sigmoid functions are used as waveshaper transfer functions to emulate the sound of analog circuitry clipping. In artificial neural networks, sometimes non-smooth functions are used instead for efficiency these are known as hard sigmoids. The van Genuchten–Gupta model is based on an inverted S-curve and applied to the response of crop yield to soil salinity.Įxamples of the application of the logistic S-curve to the response of crop yield (wheat) to both the soil salinity and depth to water table in the soil are shown in modeling crop response in agriculture. When a specific mathematical model is lacking, a sigmoid function is often used. Many natural processes, such as those of complex system learning curves, exhibit a progression from small beginnings that accelerates and approaches a climax over time. using the hyperbolic tangent mentioned above.Īpplications Inverted logistic S-curve to model the relation between wheat yield and soil salinity

The logistic curve Plot of the error functionĪ sigmoid function is a mathematical function having a characteristic "S"-shaped curve or sigmoid curve.Ī common example of a sigmoid function is the logistic function shown in the first figure and defined by the formula: σ ( x ) = 1 1 + e − x = e x e x + 1 = 1 − σ ( − x ). Mathematical function having a characteristic "S"-shaped curve or sigmoid curve


 0 kommentar(er)
0 kommentar(er)
